Specific Heat Capacity Equilibrium Temperature

Predicting the equilibrium temperature

On this page we'll dive specifically into a kind of problem that is common in thermodynamics – calculating the equilibrium temperature reached afterward two substances at different temperatures are brought into contact or (in the case of liquids and gases) mixed.

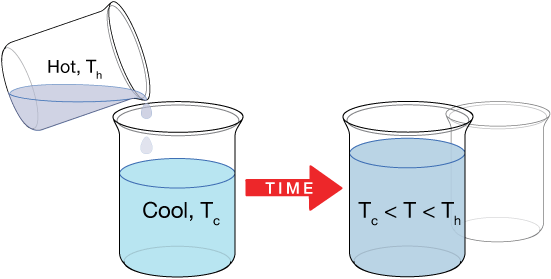
2 substances can be mixed or brought into thermal contact in whatsoever stage (liquid, solid, gas), and the equilibrium temperature will always exist somewhere between the temperatures of the starting substances. Nosotros might, for example, inquire questions like this:

2 quantities of h2o, each at a different temperature, are mixed with no loss of heat to the surroundings. Calculate the equilibrium temperature of the mixture.

Two metal objects, each at a unlike temperature and each wth a different specific oestrus, are brought into thermal contact, so that estrus can flow between them. Calculate the equilibrium temperature.

The process ever unfolds over time as shown in this graph:

The graph above shows temperature (T) vs. fourth dimension (t). When hot and cold substances are brought into contact, heat flows from the hotter substance to the colder ane. The temperature of the hot substance (T_hot) drops and the temperature of the colder substance (T_cold) rises, until they meet at the equilibrium temperature, T_eq. From the graph we tin can see the overall temperature change in each material, ΔT_hot and ΔT_{common cold} (dark-green). The starred location where the temperature bend begins to stray from the equilibrium temperature reflects what happens in most existent situations: some heat is inevitably exchanged with the surroundings and the temperature either moves upwardly or downward to establish a new equilibrium. In experiments, we'll always be concerned with T_eq.

Now let'southward practise some instance calculations to see how this works.

Newton's law of cooling

Earlier nosotros do an instance, nosotros ought to wonder why the red and bluish curves in our graph in a higher place are curved. Newton'southward law of cooling tells usa that the charge per unit of estrus transfer (which is actually the slope of those curves) is proportional to the divergence in the 2 temperatures. Notice that at the get-go of the experiment (t = 0), the transfer is rapid, but it slows down equally time goes on. This is always the way with heat transfer.

Example one

100 mL of water at 80˚C is mixed with 500 mL of water at xix˚C. Summate the equilibrium temperature of the mixture.

Solution : First we have to consider the first police force of thermodynamics, the conservation of energy. It tells us that the heat lost by the hot h2o must exactly equal the heat gained by the common cold water. This is truthful if we're working with an adiabatic system, one in which no heat is leaked to or comes in from the surroundings.

An adiabatic organisation is one in which no estrus transfer betwixt the system and the surroundings is possible. In practice, that generally means having a lot of insulation between the system (what's existence studied) and its surroundings.

This means that

$$|q_{\text{lost past hot}}| = |q_{\text{gained by cold}}|,$$

where $q$ is rut, in Joules (J). The absolute-value signs are necessary because, by long-continuing convention, heat lost by a system is negative and heat gained is positive.

Call back, also that 1 mL of water has a mass of one g (in fact, that's the definition of the gram), so 80 mL of water is 80 grams of water and and so on. It'south different for liquids of a different density.

In order to observe the equilibrium temperature, nosotros'll solve that equation, but here'south some advice first: rearrange information technology equation like this:

$$ \begin{marshal} -q_{\text{lost by hot}} &= q_{\text{gained by cold}} \\[5pt] q_{\text{lost by hot}} &+ q_{\text{gained by cold}} = 0 \tag{i} \finish{align}$$

Solving thermal equilibrium problems using equation (one) volition be much more straightforward in terms of keeping track of negative signs, which can be catchy. Here's our setup:

$$ \require{cancel} \begin{align} q_{\text{lost by hot}} + q_{\text{gained by cold}} &= 0 \\[5pt] 100 \, \cancel{g} \left(4.184 \, \frac{J}{\cancel{grand ˚C}} \right) (T_f - 80) \, \cancel{˚C} &+ \\[5pt] 500 \, \cancel{˚C} \left( 4.184 \frac{J}{\cancel{m ˚C}} \correct) (T_f - 19) \, \cancel{˚C} &= 0 \\[5pt] \terminate{align}$$

After canceling our units, we tin can now multiply, distribute, grouping terms (do our usual practiced algebra moves) and solve for $T_f :$

$$ \begin{align} 418.4 (T_f - 80) + 2092 (T_f - nineteen) &= 0 \\[5pt] 418.4 T_f - 33472 + 2092 T_f - 39748 &= 0 \\[5pt] 2510 T_f - 73220 &= 0 \stop{align}$$

$$ \begin{marshal} 2510 T_f &= 73220 \\[5pt] T_f &= 29.ii \, ˚C \terminate{align}$$

Does this make sense?

I last thing here: We should always inquire whether our solution makes sense. Is it reasonable for 80 grams of hot water to just heighten the temperature of 500 grams of nineteen˚C water past only ten degrees? That actually seems about right because the larger mass of h2o is v× the smaller ane. If the 2 masses were the same, we'd wait (because the specific heats are the same) that the equilibrium temperature would be the boilerplate of 80˚C and 19˚C, 49.five˚C.

Pro tip:

When solving for equilibrium temperatures, instead of solving $|q_{\text{lost by one substance}}| = |q_{\text{gained by the other}}|,$ it will be easier to keep runway of signs if you solve $q_{\text{lost by ane substance}} + q_{\text{gained by the other}} = 0.$

Then the merely thing you'll have to keep rails of is that $\Delta T = T_{concluding} - T_{initial}.$

Example ii: different substances

200 mL of common cold (-nineteen˚C) ethanol (C₂H_vOH, C_p = 2.46 J/g˚C, ρ = 0.789 g/mL) is added to 200 mL of liquid h2o (C_p = 4.184 J/g˚C) at 30˚C. Calculate the equilibrium temperature of the mixture.

Solution : Before yous do anything, parse the problem. Notice that we have the specific heats (C_p) of both substances, the masses (one is through the density – read on), and the initial temperatures. What nosotros're looking for is the concluding temperature, T_f.

First we need to employ the density of ethanol to find its mass:

$$200 \cancel{mL} \left( \frac{0.789 \, chiliad}{1 \, \cancel{mL}} \right) = 157.8 \, grand$$

A density calculation like this is really just a unit of measurement conversion — no big bargain. Now using our heat-alter formula $q = m C_p \Delta T,$ we can set up up our equilibrium equation, starting with the ethanol (I'll leave the units off this time for greater clarity):

Exist careful here: 200 mL is merely 200 grams for water. If you lot're doing these calculations for a different liquid substance, yous'll need its density to summate the mass from the volume before going on: volume × density = mass.

$$ \brainstorm{align} 157.8 (ii.46)(T_f - (-19)) \; &+ \\[4pt] 200 (4.184) (T_f - 30) &= 0\\[5pt] 388.19 (T_f + 19) + 836.viii (T_f - 30) &= 0 \\[5pt] 388.19 T_f + 7376 + 836.8 T_f - 25104 &= 0 \\[5pt] 1225 T_f - 17,728 &= 0 \end{align}$$

$$ \begin{align} 1225 T_f &= 17,728 \\[5pt] T_f &= 14.5 \, ˚C \end{align}$$

That makes sense. The volumes are similar, merely the ethanol can't concord as much oestrus as the water, so the temperature should terminate upwards beingness closer to the temperature of the water.

Case three: 2 metals

A 500 m block of titanium (Ti) at 650˚Cis brought into thermal contact with a ane.0 Kg (1000 yard) block of aluminum (Al) at 25˚C. The specific heats of Ti and Al are C_Ti = 0.72 J/g˚C and C_Al = 0.89 J/1000˚C. Calculate the equilibrium temperature of the blocks in one case they reach it.

Solution : Before doing our calculation, it's worth noticing that in a situation like this using solids, we tin affect the rate of heat transfer by increasing or decreasing the corporeality of surface through which heat tin can move. In the upper office of the effigy below, the ii metals touch through a smaller surface than the lower one, so we'd expect the heat transfer to be slower. Nevertheless, an equilibrium temperature will notwithstanding somewhen be reached.

To prepare upwards our calculation, we country that the rut lost by the titanium plus the heat gained past the aluminum must be equal to cypher. That'southward a statement of the police force of conservation of energy. So we have:

$$ \begin{align} 500 \cancel{m} \left(0.72 \frac{J}{\abolish{g˚C}}\right) (T_f - 650) \cancel{˚C} \; &+ \\[4pt] chiliad \cancel{g} \left(0.89 \frac{J}{\cancel{grand˚C}}\right) (T_f - 25) \cancel{˚C} &= 0 \\[5pt] 500(0.72)(T_f - 650) &+ \\[4pt] yard(0.89)(T_f - 25) &= 0 \\[5pt] 360(T_f - 650) + 890(T_f - 25) &= 0 \\[5pt] 360 T_f - 234,000 + 890 T_f - 22,250 &= 0 \\[5pt] 1,250 T_f - 256,250 &= 0 \end{align}$$

$$ \begin{align} 1,250 T_f &= 256,250 \\[5pt] T_f &= 205 \, ˚C \end{align}$$

Example 4: a alter of state

A forty m cube of water ice (yes, "water" ice – in that location are all kinds of ice) at -20˚C is dropped into 200 mL of liquid h2o at 25˚C. Assume that all of the ice melts completely. Calculate the equilibrium temperature of the water. The specific oestrus of h2o ice is C_ice = ii.05 J/g˚C and the enthalpy (latent estrus) of fusion of h2o is Δ H_f = 333.55 J/g.

Solution : We'll tackle this problem in the same way, but it's best to pause and consider what happens to the 40g of h2o (the ice) separately from the liquid, like this:

40g (common cold ice to water above 0˚C

Water ice warms from -20˚C to 0˚C, its melting temperature;

Ice undergoes a phase transition to liquid water, so the enthalpy of fusion, ΔH_f will exist required;

Resulting water at 0˚C warms to the last temperature, T_F, which is unknown.

The 200g of liquid water

200g of h2o at 25˚C cools to the same final temperature, T_F, equally above – the equilibrium temperature.

To help organize this piece of work, it sometimes helps to map out what'southward going on using function of the water heating curve, below.

The red line shows the three parts of the heating procedure for the ice, and the blue one shows the modify in the water temperature. The curves are not to scale. They come across at the equilibrium temperature, T_f.

Now adding all of these steps and setting the result equal to nada, co-ordinate to the constabulary of conservation of energy, volition go us to our concluding temperature. Here's the initial equation, without units for simplicity and with the terms in the order presented in our list to a higher place:

$$ \brainstorm{align} 40(2.05)(0 - (-twenty)) + 40(333.55) \; &+ \\[4pt] 40(4.184)(T_f - 0) + 200(four.184)(T_f - 25) &= 0 \end{align}$$

Now let's multiply, distribute, group like terms – just solid algebra moves – and solve for T_f.

$$ \begin{align} 82(xx) + 13,342 + 167 T_f + 837(T_f - 25) &= 0 \\[5pt] 1,640 + 13,342 + 167 T_f + 837 T_f - 20,925 &= 0 \\[5pt] 1004 T_f + five,943 &= 0 \\[5pt] 1004 T_f = 5,943 &\\[5pt] T_f = 5.9˚C & \end{align}$$

Does this brand sense?

Yous might call up this temperature is low in lite of our previous examples, but recall that in melting, the ice extracts an extra 333 J/grand of energy from the water without a change it its temperature. That represents an additional reduction of the h2o temperature that wasn't in those examples.

Example 5: a change of state?

A 50g cube of water ice at -20˚C is placed into 100 mL of water at 20˚C. Calculate the equilibrium temperature of the mixture.

Solution : On its surface, this is the essentially the same problem equally in instance 4. Let'due south run across how it works. The setup is the same:

$$ \begin{marshal} 50(2.05)(0 - (-20)) + fifty(333.55) \; &+ \\[4pt] 50(4.184)(T_f - 0) + 100(four.184)(T_f - 20) &= 0 \end{marshal}$$

Now to solve for the final temperature:

$$ \begin{align} 102.5(20) + 16,678 + 209.two T_f + 418.four(T_f - 20) &= 0 \\[5pt] 2,050 + 16,678 + 209.2 T_f + 418.4 T_f - viii,368 &= 0 \\[5pt] 627.vi T_f + 10,360 &= 0 \\[5pt] 627.vi T_f = -ten,360 &\\[5pt] T_f = -16.v˚C & \cease{align}$$

Does this brand sense?

No. That ice cube is unlikely to freeze the h2o to a temperature of 16˚C. In that location must exist something else going on here. Let'south take a expect at the amount of free energy stored in the liquid water above its 0˚C freezing point. That is

$$ \begin{align} q &= mC_p \Delta T \\[5pt] &= 100 \cancel{g} \left( four.184 \frac{J}{\cancel{g˚C}} \correct) (20 \cancel{˚C}) \\[5pt] &= 8,368 \, J \end{align}$$

Now compare that energy with the free energy it takes just to melt a 50 g chunk of ice at 0˚C:

$$ \begin{align} q &= g \Delta H_f = 50 \cancel{k} \left( 333.55 \frac{J}{\cancel{g˚C}} \right) \\[5pt] &= 16,678 \, J \end{align}$$

And so our h2o does non comprise enough energy to melt the ice, and we'd look to have a mixture of water and ice, coexisting at an equilibrium temperature of 0˚C, the melting temperature.

Practise problems

1.	250 mL of water (density ρ = one grand/mL, C_p = iv.184 J/g˚C) at 95˚C is added to 150 mL of ethanol (ρ = 0.789 J/yard˚C) at -four˚C. The specific heat of ethanol is 2.46 J/g˚C. Calculate the equilibrium temperature of the mixture. solution The data $$ \brainstorm{align} m_w &= 250 \, 1000 \\[5pt] C_w &= 4.184 \, J/one thousand˚C \\[5pt] T_{i,w} &= 95˚C \\[5pt] m_e &= 150 \cancel{mL} \left( \frac{0.789 \, g}{\cancel{mL}} \right) = 118.35 \, chiliad \\[5pt] C_e &= 2.46 \, J/g˚C \\[5pt] T_{i,e} &= -4˚C \end{marshal}$$ The calculation $$ \begin{align} 250(iv.184)(T_f - 95) &+ \\[4pt] 118.35(2.46)(T_f - (-4)) &= 0 \\[5pt] ane,046(T_f - 95) + 291(T_f + 4) &= 0 \\[5pt] one,337 T_f - 99,370 + 291 T_f + 1,164 &= 0 \\[5pt] ane,628 T_f - 98,206 &= 0 \end{align}$$ $$ \begin{marshal} 1,628 T_f &= 98,206 \\[5pt] T_f = \frac{98,206}{1,628} &= 60.3 ˚C \end{marshal}$$
2.	A 205 g piece of brass at 120˚C is lowered into a 300 mL h2o bath at 22˚C. The specific heats of contumely and water are C_brass = 0.360 J/thousand˚C and C_H2O = 4.184 J/g˚C, respectively. Summate the temperature of the h2o once the system has come to equilibrium. solution The data $$ \brainstorm{align} m_B &= 205 \, g \\[5pt] C_B &= 0.360 \, J/m˚C \\[5pt] T_{i,B} &= 120˚C \\[5pt] m_w &= 300 \, g \\[5pt] C_w &= 4.184 \, J/g˚C \\[5pt] T_{i,westward} &= 22˚C \end{align}$$ The calculation $$ \brainstorm{align} 205(0.360)(T_f - 120) &+ \\[4pt] 300(4.184)(T_f - 22) &= 0 \\[5pt] 73.8(T_f - 120) + 1,255(T_f - 22) &= 0 \\[5pt] 73.viii T_f - 8,856 + 1,2551 T_f - 27,610 &= 0 \\[5pt] one,329 T_f - 36,466 &= 0 \end{marshal}$$ $$ \begin{align} 1,329 T_f &= 36,466 \\[5pt] T_f = \frac{36,466}{1,329} &= 27.4 ˚C \end{marshal}$$
3.	A bar of aureate (Au) metallic of mass i.2 Kg and at 85˚C is placed in thermal contact with a 1.00 Kg block of platinum (Pt) at 900˚C. The specific heats of aureate and platinum are C_Au = 0.xiii J/g˚C and C_Pt = 0.133 J/g˚C, respectively. Calculate the equilibrium temperature of the two bars of metallic once heat has stopped flowing. Assume no loss of estrus to the surroundings. solution The data $$ \brainstorm{align} m_{Au} &= i.two \, Kg = 1,200 \, m \\[5pt] C_{Au} &= 0.13 \, J/one thousand˚C \\[5pt] T_{i,Au} &= 85˚C \\[5pt] m_{Pt} &= 1.00 \, Kg = 1,000 \, grand \\[5pt] C_{Pt} &= 0.133 \, J/g˚C \\[5pt] T_{i,Pt} &= 900˚C \stop{marshal}$$ The calculation $$ \brainstorm{align} 1,200(0.xiii)(T_f - 85) &+ \\[4pt] 1,000(0.133)(T_f - 900) &= 0 \\[5pt] 156(T_f - 85) + 133(T_f - 900) &= 0 \\[5pt] 156 T_f - thirteen,260 + 133 T_f - 119,700 &= 0 \\[5pt] 289 T_f - 132,960 &= 0 \end{align}$$ $$ \begin{align} 289 T_f &= 132,960 \\[5pt] T_f = \frac{132,960}{289} &= 460 ˚C \cease{align}$$
four.	82 grams of gilded metallic (C_p = 0.13 J/grand˚C) at 1200˚C is poured into an 800g steel mold which is at an initial temperature of 22˚C. The specific oestrus of steel is 0.466 J/g˚C and the enthalpy (latent rut) of fusion of gold is ΔH_f = 67 J/one thousand. The melting temperature of aureate is 1064˚C. Calculate the equilibrium temperature of the gold. Volition the gold solidify right away? solution The information $$ \begin{align} m_{Au} &= 82 \, g \\[5pt] C_{Au} &= 0.thirteen \, J/g˚C \\[5pt] T_{i,Au} &= one,200˚C \\[5pt] m_{steel} &= 800 \, grand \\[5pt] C_{steel} &= 0.466 \, J/g˚C \\[5pt] T_{i,steel} &= 22˚C \end{align}$$ The calculation $$ \begin{align} 82(0.13)(T_f - 1200) &+ \\[4pt] 800(0.466)(T_f - 22) &= 0 \\[5pt] 10.66(T_f - 1200) + 372.eight(T_f - 22) &= 0 \\[5pt] 10.66 T_f - 12,792 + 372.8 T_f - 8,201.half-dozen &= 0 \\[5pt] 383.46 T_f - 132,960 &= 0 \terminate{marshal}$$ $$ \begin{align} 383.46 T_f &= 20,994 \\[5pt] T_f = \frac{20,994}{383.46} &= 54.7 ˚C \end{align}$$
5.	An 25 g chunk of ice at -18˚C is dropped into an insulated beaker containing 500 grand of methanol at 19˚C. The specific heats of ice and water are C_ice = 2.05 J/g˚C and C_methanol = 2.53 J/g˚C, respectively. Summate the equilibrium temperature of the mixture. Assume that all of the ice melts. solution The data $$ \begin{marshal} m_{ice} &= 25 \, thou \\[5pt] C_{ice} &= 2.05 \, J/g˚C \\[5pt] T_{i,ice} &= -18˚C \\[5pt] m_{methanol} &= 500 \, yard \\[5pt] C_{steel} &= 2.53 \, J/1000˚C \\[5pt] T_{i,steel} &= 19˚C \end{align}$$ The calculation $$ \brainstorm{align} 25(2.05)(T_f -(-18)) &+ \\[4pt] 500(2.53)(T_f - nineteen) &= 0 \\[5pt] 51.25(T_f + 18) + 1265(T_f - 19) &= 0 \\[5pt] 51.25 T_f + 922.5 + 1265 T_f - 24,035 &= 0 \\[5pt] 1316 T_f - 23,112 &= 0 \end{align}$$ $$ \brainstorm{align} 1316 T_f &= 23,112 \\[5pt] T_f = \frac{23,112}{1316} &= 17.six ˚C \stop{align}$$